Archimedes was born about 287 BCE and died during the sack of Syracuse by the Romans in 212 BCE. He is considered the greatest mathematician, physicist, and engineer of antiquity. His emphasis on using both mathematical models and experiments in investigating nature exerted a fundamental influence on the founders of modern mechanics, such as Galileo and Newton. He invented the hydraulic screw and is credited with the explanation of the principle of the lever, as well as the principle of the buoyancy of liquids. He regarded the relationship between the surface and volume of a sphere and its circumscribing cylinder as his greatest discovery.
His books were translated into Arabic and from Arabic into Latin during the Middle Ages, especially by William of Moerbeke (1215-1286). The first Latin (partial) edition published in Venice by the mathematician Niccolo’ Tartaglia (1500-1557) was based on Moerbeke’s translation, and was followed in 1544 by a Greek and Latin edition published in Basel by Thomas Geschauff (Lat. Venatorius 1490–1551) and by a more accurate Latin translation by Federico Commandino (Venice, 1558). The present text, the 1615 Latin and Greek edition of the Opera quae extant translated by David Rivault de Fleurence (1571–1616) and printed in Paris by Claude Morel, was extremely influential, also due to its nice illustrations.
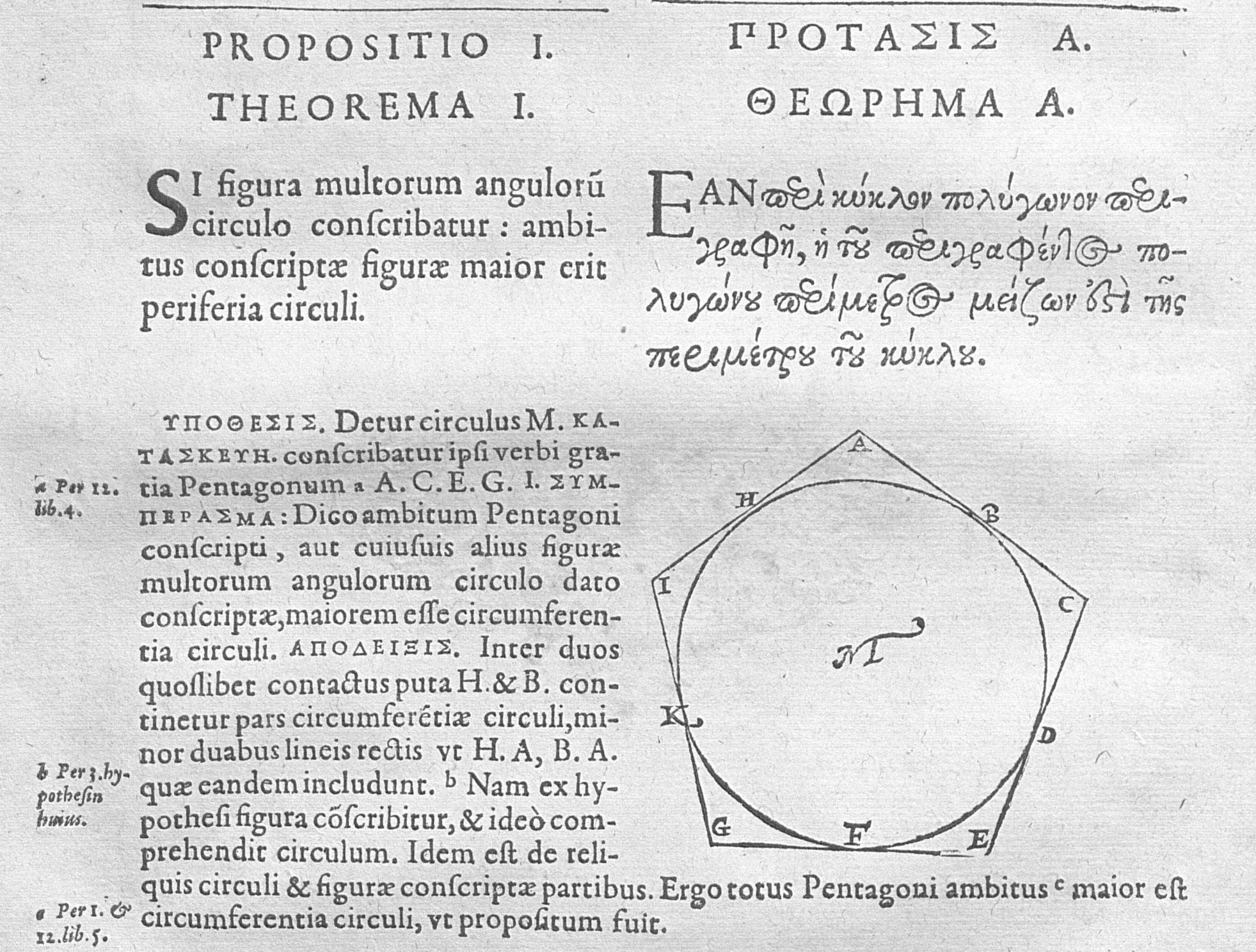
The figure here shows the diagram illustrating theorem I of book I of Archimedes’ treatise On the Sphere and the Cylinder, with Latin and Greek text side by side. It asserts that if a polygon is circumscribed around a circle, the perimeter of the circumscribed polygon is greater than the perimeter of the circle. The theorem is demonstrated in the case of a pentagon, starting from the segment AHB at the top and proceeding exhaustively towards the bottom. Compared with Euclides’ long and explicit axiomatic demonstrations, this brief and intuitive demonstration by Archimedes relies on visual clues (the letters are not defined, as in Euclid, but simply assumed from the related diagram) and on the implicit application of axioms and definitions, such as the general property of concavity in this case, defined above (but not explicitly invoked in the demonstration).
Sources- Wikipedia
- Archimedes, Reviel Netz. The Works of Archimedes, The Two Books On the Sphere and the Cylinder. Translation and Commentary. Cambridge University Press (2004), Volume 1, pp. 41-43.
- George Sarton, Introduction to the history of science, Baltimore: Williams & Wilkins Co., 1927-1948, vol. 1, pp. 169-172.
- Ludovico Geymonat (ed.) Storia del pensiero filosofico e scientifico. Milano, Garzanti, 1970-1972, vol. 1 pp. 250-253.
- Paul T Keyser and Georgia Irby-Massie (eds), The encyclopedia of ancient natural scientists : the Greek tradition and its many heirs, Milton Park, Abingdon, Oxon ; New York, NY : Routledge, 2008.
- http://exhibits.museogalileo.it/archimedes/object/ArchimedesArchimedisOperaQuaeExtantEditedByRivault.html
- http://peenef2.republika.pl/angielski/hasla/a/archimedes.html
- On Rivault’s translation: http://www.sil.si.edu/libraries/Dibner/newacq.htm